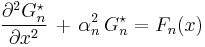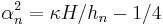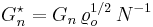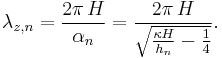Atmospheric tide
Atmospheric tides are global-scale periodic oscillations of the atmosphere. In many ways they are analogous to ocean tides. Atmospheric tides can be excited by:
- The regular day/night cycle in the insolation of the atmosphere
- The gravitational field pull of the Moon
- Non-linear interactions between tides and planetary waves.
- Large-scale latent heat release due to deep convection in the tropics.
Contents |
General characteristics
The largest-amplitude atmospheric tides are mostly generated in the troposphere and stratosphere when the atmosphere is periodically heated as water vapour and ozone absorb solar radiation during the day. The tides generated are then able to propagate away from these source regions and ascend into the mesosphere and thermosphere. Atmospheric tides can be measured as regular fluctuations in wind, temperature, density and pressure. Although atmospheric tides share much in common with ocean tides they have two key distinguishing features:
- Atmospheric tides are primarily excited by the Sun's heating of the atmosphere whereas ocean tides are excited by the Moon's gravitational pull and to a lesser extent by the Sun's gravity. This means that most atmospheric tides have periods of oscillation related to the 24-hour length of the solar day whereas ocean tides have periods of oscillation related both to the solar day as well as to the longer lunar day (time between successive lunar transits) of about 24 hours 51 minutes.
- Atmospheric tides propagate in an atmosphere where density varies significantly with height. A consequence of this is that their amplitudes naturally increase exponentially as the tide ascends into progressively more rarefied regions of the atmosphere (for an explanation of this phenomenon, see below). In contrast, the density of the oceans varies only slightly with depth and so there the tides do not necessarily vary in amplitude with depth.
At ground level, atmospheric tides can be detected as regular but small oscillations in surface pressure with periods of 24 and 12 hours. However, at greater heights the amplitudes of the tides can become very large. In the mesosphere (heights of ~ 50–100 km) atmospheric tides can reach amplitudes of more than 50 m/s and are often the most significant part of the motion of the atmosphere.
The reason for this dramatic growth in amplitude from tiny fluctuations near the ground to oscillations that dominate the motion of the mesosphere lies in the fact that the density of the atmosphere decreases with increasing height. As tides or waves propagate upwards, they move into regions of lower and lower density. If the tide or wave is not dissipating, then its kinetic energy density must be conserved. Since the density is decreasing, the amplitude of the tide or wave increases correspondingly so that energy is conserved. The amplitude of a wave at a height of z can thus be described by the equation:
where 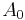 is the initial amplitude of the wave,
is the initial amplitude of the wave, 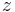 is height and
is height and  is the scale height of the atmosphere.
is the scale height of the atmosphere.
Following this growth with height atmospheric tides have much larger amplitudes in the middle and upper atmosphere than they do at ground level.
Solar atmospheric tides
The largest amplitude atmospheric tides are generated by the periodic heating of the atmosphere by the Sun – the atmosphere is heated during the day and not heated at night. This regular diurnal (daily) cycle in heating generates tides that have periods related to the solar day. It might initially be expected that this diurnal heating would give rise to tides with a period of 24 hours, corresponding to the heating's periodicity. However, observations reveal that large amplitude tides are generated with periods of 24 and 12 hours. Tides have also been observed with periods of 8 and 6 hours, although these latter tides generally have smaller amplitudes. This set of periods occurs because the solar heating of the atmosphere occurs in an approximate square wave profile and so is rich in harmonics. When this pattern is decomposed into separate frequency components using a fourier transform, as well as the mean and daily (24-hr) variation, significant oscillations with periods of 12, 8 and 6 hrs are produced. Tides generated by the gravitational effect of the sun are very much smaller than those generated by solar heating. Solar tides will refer to only thermal solar tides from this point.
Solar energy is absorbed throughout the atmosphere some of the most significant in this context are water vapor at (~0–15 km) in the troposphere, ozone at (~30 to 60 km) in the stratosphere and molecular oxygen and molecular nitrogen at (~120 to 170 km) in the thermosphere. Variations in the global distribution and density of these species results in changes in the amplitude of the solar tides. The tides are also affected by the environment through which they travel.
Solar tides can be separated into two components: migrating and non-migrating.
Migrating solar tides
Migrating tides are sun synchronous – from the point of view of a stationary observer on the ground they propagate westwards with the apparent motion of the sun. As the migrating tides stay fixed relative to the sun a pattern of excitation is formed that is also fixed relative to the Sun. Changes in the tide observed from a stationary viewpoint on the Earth's surface are caused by the rotation of the Earth with respect to this fixed pattern. Seasonal variations of the tides also occur as the Earth tilts relative to the Sun and so relative to the pattern of excitation.[1]
The migrating solar tides have been extensively studied both through observations and mechanistic models.[2]
Non-migrating solar tides
Non-migrating tides can be thought of as global-scale waves with the same periods as the migrating tides. However, non-migrating tides do not follow the apparent motion of the sun. Either they do not propagate horizontally, they propagate eastwards or they propagate westwards at a different speed to the sun. These non-migrating tides may be generated by differences in topography with longitude, land-sea contrast and surface interactions. An important source is latent heat release due to deep convection in the tropics.
The primary source for the 24-hr tide is in the lower atmosphere where surface effects are important. This is reflected in a relatively large non-migrating component seen in longitudinal differences in tidal amplitudes. Largest amplitudes have been observed over South America, Africa and Australia.[3]
Lunar atmospheric tides
Atmospheric tides are also produced through the gravitational effects of the Moon.[4] Lunar (gravitational) tides are much weaker than solar (thermal) tides and are generated by the motion of the Earth's oceans (caused by the Moon) and to a lesser extent the effect of the Moon's gravitational attraction on the atmosphere.
Classical tidal theory
The basic characteristics of the atmospheric tides are described by the classical tidal theory.[5] By neglecting mechanical forcing and dissipation, the classical tidal theory assumes that atmospheric wave motions can be considered as linear perturbations of an initially motionless zonal mean state that is horizontally stratified and isothermal. The two major results of the classical theory are
- atmospheric tides are eigenmodes of the atmosphere described by Hough functions
- amplitudes grow exponentially with height.
Basic equations
The primitive equations lead to the linearized equations for perturbations (primed variables) in a spherical isothermal atmosphere:[6]
- horizontal momentum equations
- energy equation
- continuity equation
with the definitions
 eastward zonal wind
eastward zonal wind northward meridional wind
northward meridional wind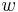 upward vertical wind
upward vertical wind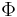 geopotential,
geopotential, 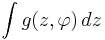
 square of Brunt-Vaisala (buoyancy) frequency
square of Brunt-Vaisala (buoyancy) frequency angular velocity of the Earth
angular velocity of the Earth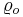 density
density 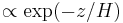
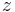 altitude
altitude geographic longitude
geographic longitude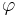 geographic latitude
geographic latitude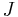 heating rate per unit mass
heating rate per unit mass radius of the Earth
radius of the Earth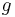 gravity acceleration
gravity acceleration constant scale height
constant scale height time
time
Separation of variables
The set of equations can be solved for atmospheric tides, i.e., longitudinally propagating waves of zonal wavenumber 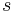 and frequency
and frequency 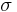 . Zonal wavenumber
. Zonal wavenumber 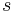 is a positive integer so that positive values for
is a positive integer so that positive values for 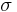 correspond to eastward propagating tides and negative values to westward propagating tides. A separation approach of the form
correspond to eastward propagating tides and negative values to westward propagating tides. A separation approach of the form
and doing some math [7] yields expressions for the latitudinal and vertical structure of the tides.
Laplace's tidal equation
The latitudinal structure of the tides is described by the horizontal structure equation which is also called Laplace's tidal equation:
with Laplace operator
using 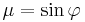 ,
, 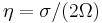 and eigenvalue
and eigenvalue
Hence, atmospheric tides are eigenoscillations (eigenmodes)of Earth's atmosphere with eigenfunctions 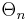 , called Hough functions, and eigenvalues
, called Hough functions, and eigenvalues 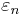 . The latter define the equivalent depth
. The latter define the equivalent depth 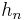 which couples the latitudinal structure of the tides with their vertical structure.
which couples the latitudinal structure of the tides with their vertical structure.
Vertical structure equation
For bounded solutions and at altitudes above the forcing region, the vertical structure equation in its canonical form is:
with solution
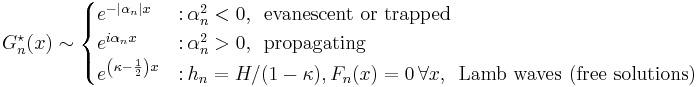
using the definitions
Propagating solutions
Therefore, each wavenumber/frequency pair (a tidal component) is a superposition of associated Hough functions (often called tidal modes in the literature) of index n. The nomenclature is such that a negative value of n refers to evanescent modes (no vertical propagation) and a positive value to propagating modes. The equivalent depth 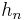 is linked to the vertical wavelength
is linked to the vertical wavelength 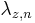 , since
, since  is the vertical wavenumber:
is the vertical wavenumber:
For propagating solutions 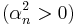 , the vertical group velocity
, the vertical group velocity
becomes positive (upward energy propagation) only if 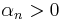 for westward
for westward 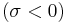 or if
or if 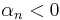 for eastward
for eastward  propagating waves. At a given height
propagating waves. At a given height 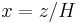 , the wave maximizes for
, the wave maximizes for
For a fixed longitude  , this in turn always results in downward phase progression as time progresses, independent of the propagation direction. This is an important result for the interpretation of observations: downward phase progression in time means an upward propagation of energy and therefore a tidal forcing lower in the atmosphere. Amplitude increases with height
, this in turn always results in downward phase progression as time progresses, independent of the propagation direction. This is an important result for the interpretation of observations: downward phase progression in time means an upward propagation of energy and therefore a tidal forcing lower in the atmosphere. Amplitude increases with height 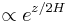 , as density decreases.
, as density decreases.
Dissipation
Damping of the tides occurs primarily in the lower thermosphere region, and may be caused by turbulence from breaking gravity waves. A similar phenomena to ocean waves breaking on a beach, the energy dissipates into the background atmosphere. Molecular diffusion also becomes increasingly important at higher levels in the lower thermosphere as the mean free path increases in the rarefied atmosphere.
Effects of atmospheric tide
The tides form an important mechanism for transporting energy input into the lower atmosphere from the upper atmosphere, while dominating the dynamics of the mesosphere and lower thermosphere. Therefore, understanding the atmospheric tides is essential in understanding the atmosphere as a whole. Modeling and observations of atmospheric tides are needed in order to monitor and predict changes in the Earth's atmosphere.
See also
Notes and references
- ^ Global Scale Wave Model UCAR
- ^ GSWM References
- ^ Hagan, M.E., J.M. Forbes and A. Richmond, 2003: Atmospheric Tides, Encyclopedia of Atmospheric Sciences
- ^ "Tides found in atmosphere", Sydney Morning Herald, September 9, 1947, http://news.google.com/newspapers?id=X30QAAAAIBAJ&sjid=wZMDAAAAIBAJ&pg=3035,1211515&dq=lyle-medal&hl=en.
- ^ Chapman, S., and R. S. Lindzen, Atmospheric Tides, D. Reidel, Norwell, Mass., 1970.
- ^ Holton, J. R., The Dynamic Meteorology of the Stratosphere and Mesosphere, Meteor. Monog., 15(37), American Meteorological Society, MA, 1975.
- ^ J. Oberheide, On large-scale wave coupling across the stratopause, Appendix A2, pp 113–117, University of Wuppertal, 2007.
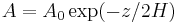
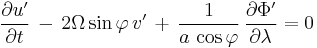
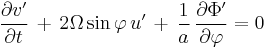
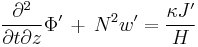
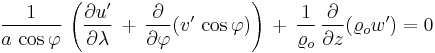

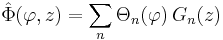
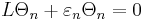
![{L}=\frac{\partial}{\partial \mu} \left[ \frac{(1-\mu^2)}{(\eta^2 - \mu^2)} \,
\frac{\partial}{\partial \mu} \right] - \frac{1}{\eta^2 - \mu^2} \,
\left[ -\frac{s}{\eta} \, \frac{(\eta^2 %2B \mu^2)}{(\eta^2 - \mu^2)} %2B
\frac{s^2}{1-\mu^2} \right]](/2012-wikipedia_en_all_nopic_01_2012/I/cfbb002466a8e01010d08e5e34dddf45.png)